
But it just oscillatesīetween these two values. Infinity or negative infinity or something like that. Or another way to thinkĪbout it, the limit as n approaches infinity To grow much faster than the denominator. So for the same reasonĪs the b sub n sequence, this thing is going to diverge. Now let's look at thisĭegree in the numerator than we have in the denominator. Have this as 100, e to the 100th power is a But the giveaway is thatĪnd the denominator. To be approaching n squared over n squared, or 1. To grow anywhere near as fast as the n squared terms,Įspecially for large n's. Numerator- this term is going to represent most of the value. Really, really large, what dominates in the Think about it is n gets really, really, really, Numerator and the denominator and figure that out. Growing faster, in which case this might converge to 0? Or maybe they're growingĪt the same level, and maybe it'll converge Is going to go to infinity and this thing's And we care about the degreeīecause we want to see, look, is the numerator growingįaster than the denominator? In which case this thing What's happening as n gets larger and larger is lookĪt the degree of the numerator and the degree of
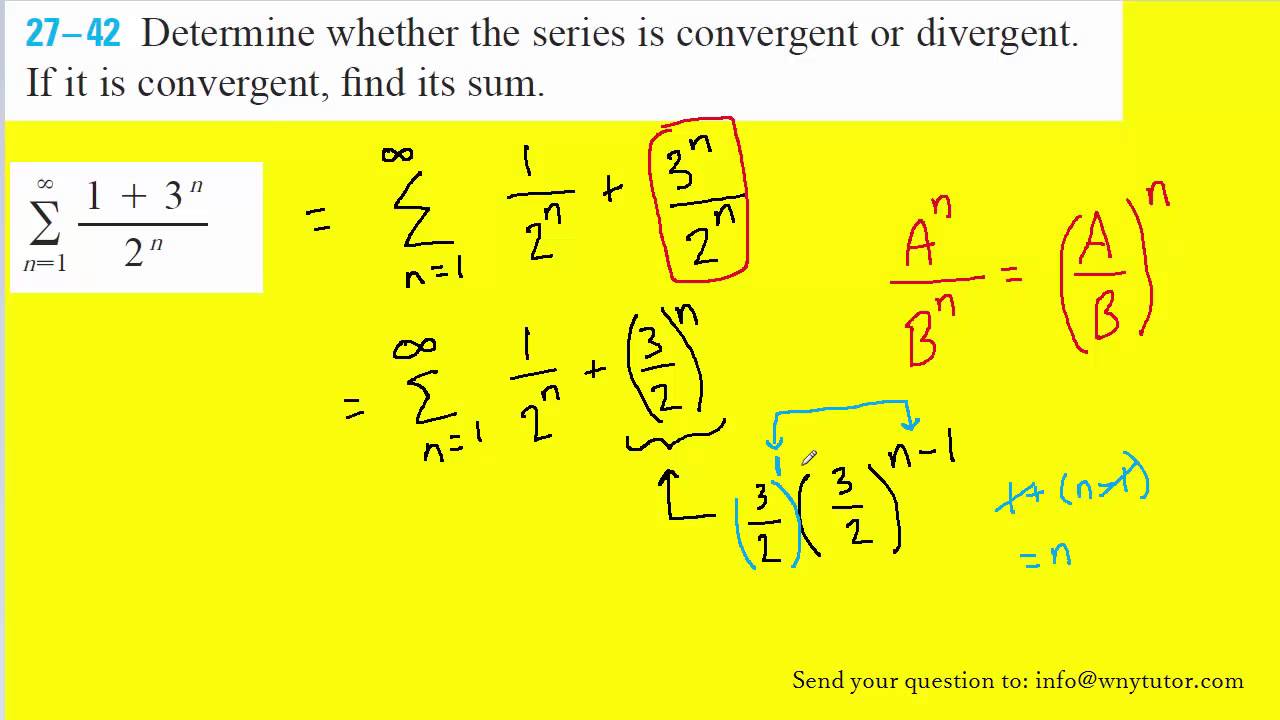
SUM OF GEOMETRIC SEQUENCE CONVERGE PLUS
N plus 1, the denominator n times n minus 10. To pause this video and try this on your ownīefore I'm about to explain it. Larger and larger, that the value of our sequence You to think about is whether these sequencesĬonverge just means, as n gets larger and
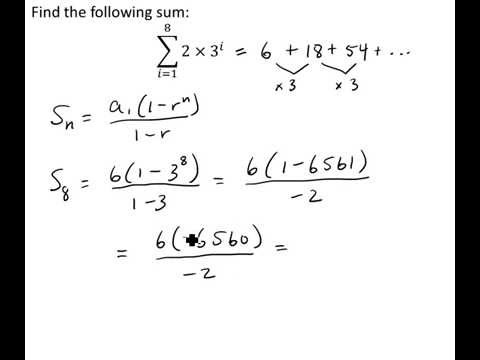
SUM OF GEOMETRIC SEQUENCE CONVERGE SERIES
In other words, a conditionally convergent series has the property that you get different answers if you rearrange or regroup the terms.įour different sequences here. Thus, conditionally convergent series are quite difficult to work with and it is very easy to get nonsense answers that look like they are correct. Thus, it is possible (by using the associative property and/or the commutative property) to group the terms of a conditionally convergent series to make it look like the series converges to any arbitrarily chosen number or to make it look like the the series diverges. The commutative and associative properties do not hold for conditionally convergent series. The sum of its negative terms diverges to negative infinity. The sum of its positive terms diverges to positive infinity.ĥ. It has both positive and negative terms.Ĥ. The series is convergent, that is it approaches a finite sum.ģ. Their "proof" was utter nonsense.Ī series is defined to be conditionally convergent if and only if it meets ALL of these requirements:Ģ.
Thus when S fails to exist, it is possible to get various nonsensical and contradictory solutions.īasic mathematical operations all require that S exists, if S does not exist the operations can still produce "answers" but they will be nonsense.īTW, the Numberphile video where they "proved" that S of the positive integers was -1/12 made use of such nonsense with divergent series. Since it is possible to have multiple contradictory sums for S, it must be the case that S fails to exist. And, for that matter, it does not hold that S + S = 2S.
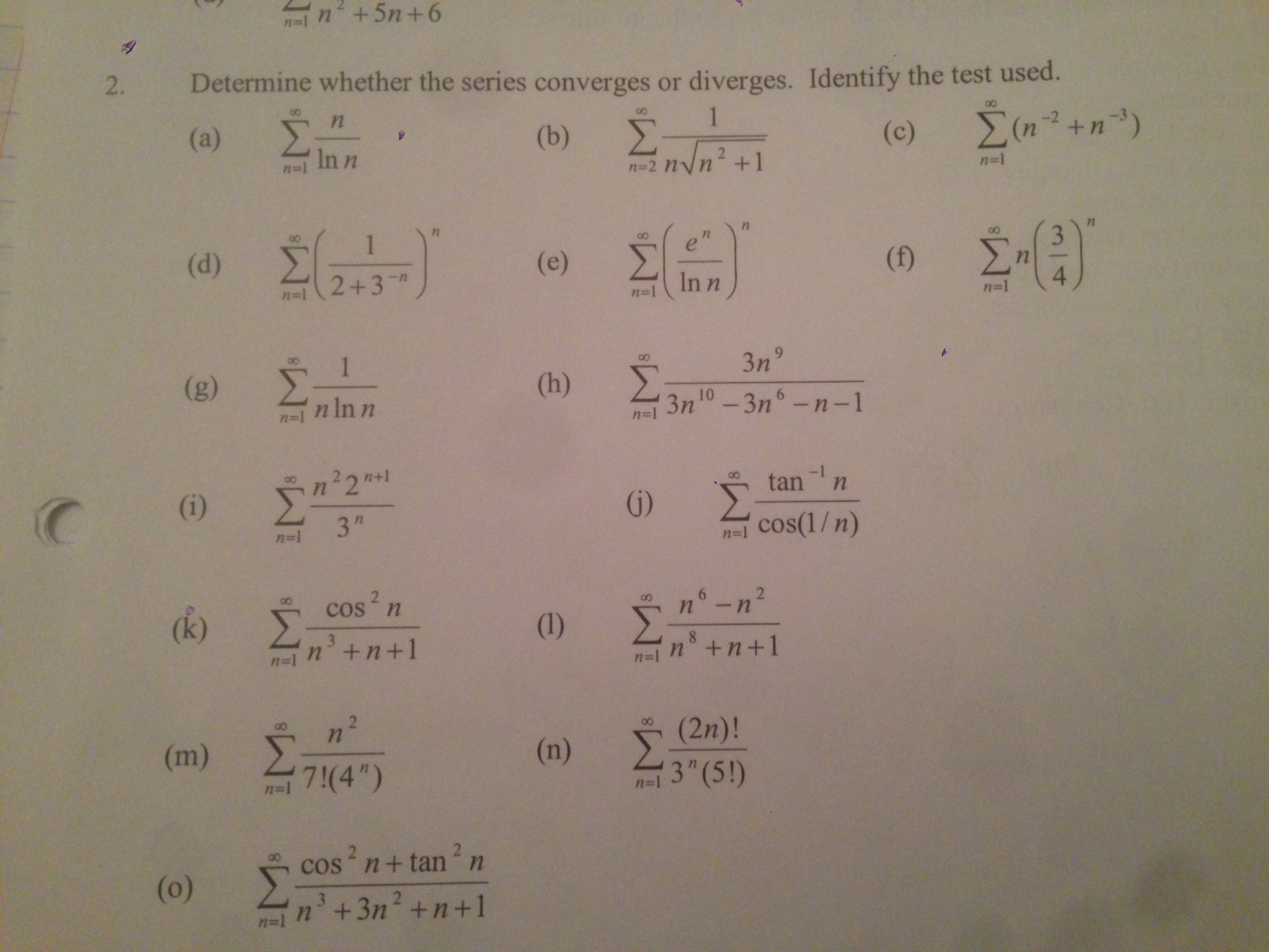
It is not the case that the associative property holds for this particular series. It Is NOT the case that S=½ nor any of the other values we could come up with.

This is obviously absurd and self-contradictory. So let us say that I added S to itself 999 times, giving me 1000S = S. It is still 1-1+1-1+1-1.Īnd, of course, I can add as many sets of S to each other as a like and they will still be the same sum, they will still be 1-1+1-1+1-1. Notice that if I add another copy of the sum 1-1+1-1+1-1. By assumption: size z < 1 So size z fulfils the. It remains to demonstrate absolute convergence: The absolute value of size z is just size z. They have no more meaning than the "proofs" 1=2 which contain a hidden division by zero. 1.1 Corollary 1 1.2 Corollary 2 2 Proof 1 3 Proof 2 4 Proof 3 5 Proof 4 6. In short, S fails to exist for a divergent series, thus computations with S are meaningless. Retrieved May 16, 2005.You cannot assume the associative property applies to an infinite series, because it may or may not hold. "Series", Encyclopedia of Mathematics, EMS Press, 2001.More precisely, an infinite sequence ( a 0, a 1, a 2, … ) See also In mathematics, a series is the sum of the terms of an infinite sequence of numbers. For other uses, see Convergence (disambiguation). "Convergence (mathematics)" redirects here.


 0 kommentar(er)
0 kommentar(er)
